Problems with the Electroweak 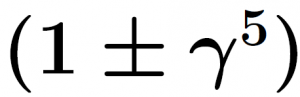 Factor
Factor
The following discussion relies on these principles:
- The primary objective of a physical theory is to provide an acceptable prediction and interpretation of experimental results that are carried out within its domain of validity.
- Quantum mechanics uses a wave-function and operators. An application of an appropriate operator to the wave function that represents a quantum system, yields the required quantity.
- There is a correspondence between quantum mechanics (QM) and quantum field theory (QFT) (see [1], p. 49).
- The Hilbert space is a crucial element of QM (see [1], pp. 49, 50). The Fock space of QFT is an extension of a Hilbert space of QM [2].
- A quantum theory of a specific interaction relies on an appropriate Lagrangian density. The electroweak Lagrangian density contains the factor
 which operates on a Dirac spinor (see [4], p. 308). The following analysis uses the principles 1-5 for an examination of the validity of a more general factor
which operates on a Dirac spinor (see [4], p. 308). The following analysis uses the principles 1-5 for an examination of the validity of a more general factor 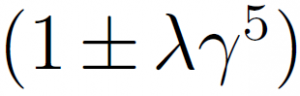 , where
, where 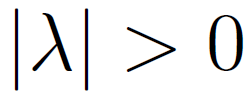 is a pure number.
is a pure number.
Let us see what happens after applying ![]() to the function of a free motionless spin-up Dirac spinor
to the function of a free motionless spin-up Dirac spinor
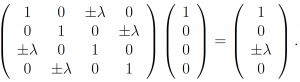
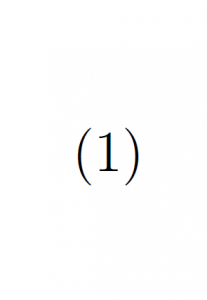
Here the notation of the ![]() matrices is that of [5], p. 17.
matrices is that of [5], p. 17.
Let us examine the three cases where ![]() .
.
- If
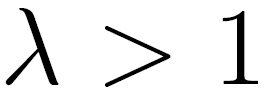 then the right-hand side of (1) is a negative-energy Dirac spinor (see [5], pp. 28-30). It means that in this case the operator
then the right-hand side of (1) is a negative-energy Dirac spinor (see [5], pp. 28-30). It means that in this case the operator 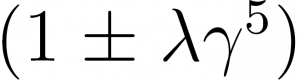 projects a massive motionless Dirac particle into an unphysical state. This state is not included in the Hilbert space and expectation values of observables cannot be calculated.
projects a massive motionless Dirac particle into an unphysical state. This state is not included in the Hilbert space and expectation values of observables cannot be calculated. - If
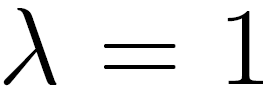 then the right-hand side of (1) is a Dirac spinor that has an infinite energy-momentum (see [5], p. 30). It means that the operator
then the right-hand side of (1) is a Dirac spinor that has an infinite energy-momentum (see [5], p. 30). It means that the operator 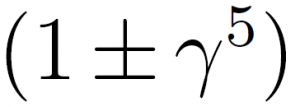 projects a massive motionless Dirac particle into an unphysical state and the previous result holds.
projects a massive motionless Dirac particle into an unphysical state and the previous result holds. 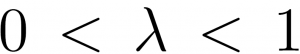 then the right-hand side of (1) is a Dirac spinor that moves in the z-direction. The energy of this spinor is greater than that of the original motionless spinor. It follows that in this case the operator
then the right-hand side of (1) is a Dirac spinor that moves in the z-direction. The energy of this spinor is greater than that of the original motionless spinor. It follows that in this case the operator 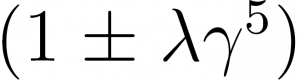 violates energy conservation.
violates energy conservation.
Conclusion: The electroweak factor ![]() is unacceptable.
is unacceptable.
Research topic: Can the above mentioned discrepancy of the electroweak theory be resolved?
References
[1] S.Weinberg, The Quantum Theory of Fields, Vol. I (Cambridge University Press, Cambridge, 1995).
[2] https://en.wikipedia.org/wiki/Fock_space
[3] R. P. Feynman and M. Gell-Mann, Phys. Rev. 109, 193 (1958).
[4] S. Weinberg, The Quantum Theory of Fields, Vol. II (Cambridge University Press, Cambridge, 1995).
[5] J. D. Bjorken and S. D. Drell, Relativistic Quantum Mechanics (McGraw-Hill, New York, 1964).
